B10CDF

B10CDF (LET)

Library Function

Compute the Burr type 10 cumulative distribution function with shape parameter r.

The Burr type 10 distribution is typically defined in terms of its cumulative distribution function:

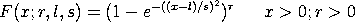
with r, l, and s denoting the shape, location, and scale parameters, respectively.

The case where l = 0 and s = 1 is referred to as the standard Burr type 10 distribution.

If r = 1, the Burr type 10 distribution is equivalent to the Rayleigh distribution. For this reason, the Burr type 10 distribution is also referred to as the generalized Rayleigh distribution. It has found use in reliability applications.

LET <y> = B10CDF(<x>,<r>,<loc>,<scale>)
                        <SUBSET/EXCEPT/FOR qualification>
where <x> is a number, parameter, or variable;
            <y> is a variable or a parameter (depending on what <x> is) where the computed Burr type 10 cdf value is stored;
            <r> is a positive number, parameter, or variable that specifies the shape parameter;
            <loc> is a number, parameter, or variable that specifies the location parameter;
            <scale> is a positive number, parameter, or variable that specifies the scale parameter;
and where the <SUBSET/EXCEPT/FOR qualification> is optional.

If <loc> and <scale> are omitted, they default to 0 and 1, respectively.

LET A = B10CDF(0.3,0.2)
LET Y = B10CDF(X,0.5,0,5)
PLOT B10CDF(X,2,0,3) FOR X = 0 0.01 5

None

BURR TYPE X is a synonym for BURR TYPE 10.

B10PDF	= Compute the Burr type 10 probability density function.
B10PPF	= Compute the Burr type 10 percent point function.
BU2PDF	= Compute the Burr type 2 probability density function.
BU3PDF	= Compute the Burr type 3 probability density function.
BU4PDF	= Compute the Burr type 4 probability density function.
BU5PDF	= Compute the Burr type 5 probability density function.
BU5PDF	= Compute the Burr type 6 probability density function.
BU7PDF	= Compute the Burr type 7 probability density function.
BU8PDF	= Compute the Burr type 8 probability density function.
BU9PDF	= Compute the Burr type 9 probability density function.
B11PDF	= Compute the Burr type 11 probability density function.
B12PDF	= Compute the Burr type 12 probability density function.
RAYPDF	= Compute the Rayleigh probability density function.
WEIPDF	= Compute the Weibull probability density function.
EWEPDF	= Compute the exponentiated Weibull probability density function.

Burr (1942), "Cumulative Frequency Functions", Annals of Mathematical Statistics, 13, pp. 215-232.

Raqab and Kundu (2006), "Burr Type X Distributions: Revisited", Journal of Probability and Statistical Sciences, Vol. 4, No. 2, pp. 179-193.

Johnson, Kotz, and Balakrishnan (1994), "Contiunuous Univariate Distributions--Volume 1", Second Edition, Wiley, pp. 53-54.

Devroye (1986), "Non-Uniform Random Variate Generation", Springer-Verlang, pp. 476-477.

Distributional Modeling

2007/10

 
LABEL CASE ASIS
TITLE CASE ASIS
TITLE OFFSET 2
.
MULTIPLOT 2 2
MULTIPLOT CORNER COORDINATES 0 0 100 95
MULTIPLOT SCALE FACTOR 2
.
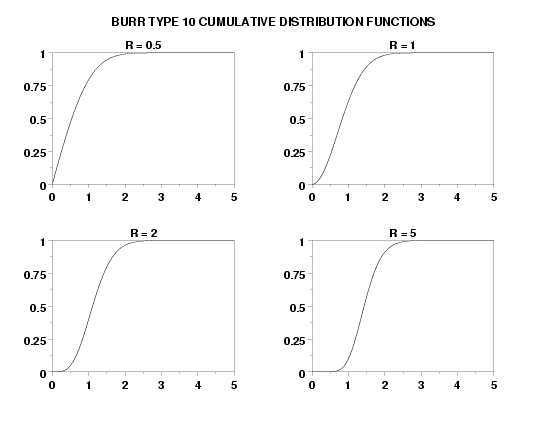
LET R  = 0.5
TITLE R = ^r
PLOT B10CDF(X,R) FOR X = 0.01  0.01  5
.
LET R  = 1
TITLE R = ^r
PLOT B10CDF(X,R) FOR X = 0.01  0.01  5
.
LET R  = 2
TITLE R = ^r
PLOT B10CDF(X,R) FOR X = 0.01  0.01  5
.
LET R  = 5
TITLE R = ^r
PLOT B10CDF(X,R) FOR X = 0.01  0.01  5
.
END OF MULTIPLOT
.
JUSTIFICATION CENTER
MOVE 50 97
TEXT Burr Type 10 Cumulative Distribution Functions