5. Process Improvement
5.6. Case Studies
5.6.1. Eddy Current Probe Sensitivity Case Study

5.6.1.2.

Initial Plots/Main Effects

Plot the Data: Ordered Data Plot

The first step in the analysis is to generate an ordered data plot.

Conclusions from the Ordered Data Plot

We can make the following conclusions based on the ordered data plot.

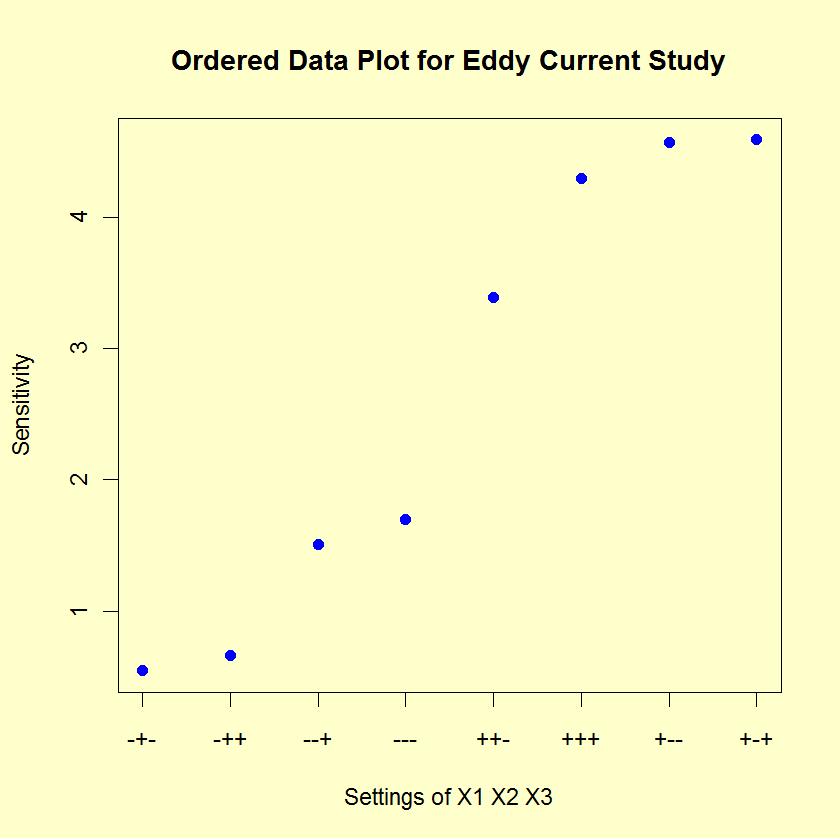
1. Important Factors: The four highest response values have X1 = + while the four lowest response values have X1 = -. This implies X1 is the most important factor. When X1 = -, the - values of X2 are higher than the + values of X2. Similarly, when X1 = +, the - values of X2 are higher than the + values of X2. This implies X2 is important, but less so than X1. There is no clear pattern for X3.

2. Best Settings: In this experiment, we are using the device as a detector, and so high sensitivities are desirable. Given this, our first pass at best settings yields (X1 = +1, X2 = -1, X3 = either).

Plot the Data: DOE Scatter Plot

The next step in the analysis is to generate a DOE scatter plot.

Conclusions from the DOE Scatter Plot

We can make the following conclusions based on the DOE scatter plot.

1. Important Factors: X1 (Number of Turns) is clearly important. When X1 = -1, all four senstivities are low, and when X1 = +1, all four sensitivities are high. X2 (Winding Distance) is less important. The four sensitivities for X2 = -1 are slightly higher, as a group, than the four sensitivities for X2 = +1. X3 (Wire Gauge) does not appear to be important at all. The sensitivity is about the same (on the average) regardless of the settings for X3.

2. Best Settings: In this experiment, we are using the device as a detector, so high sensitivities are desirable. Given this, our first pass at best settings yields (X1 = +1, X2 = -1, X3 = either).

3. There does not appear to be any significant outliers.

Check for Main Effects: DOE Mean Plot

One of the primary questions is: what are the most important factors? The ordered data plot and the DOE scatter plot provide useful summary plots of the data. Both of these plots indicated that X1 is clearly important, X2 is somewhat important, and X3 is probably not important.

The DOE mean plot shows the main effects. This provides probably the easiest to interpret indication of the important factors.

Conclusions from the DOE Mean Plot

The DOE mean plot (or main effects plot) reaffirms the ordering of the DOE scatter plot, but additional information is gleaned because the eyeball distance between the mean values gives an approximation to the least-squares estimate of the factor effects.

We can make the following conclusions from the DOE mean plot.

1. Important Factors:
   X1 (effect = large: about 3 ohms)
   X2 (effect = moderate: about -1 ohm)
   X3 (effect = small: about 1/4 ohm)
   

2. Best Settings: As before, choose the factor settings that (on the average) maximize the sensitivity:
   (X1,X2,X3) = (+,-,+)

Comparison of Plots

All of these plots are used primarily to detect the most important factors. Because it plots a summary statistic rather than the raw data, the DOE mean plot shows the main effects most clearly. However, it is still recommended to generate either the ordered data plot or the DOE scatter plot (or both). Since these plot the raw data, they can sometimes reveal features of the data that might be masked by the DOE mean plot.