5. Process Improvement
5.5. Advanced topics
5.5.4. What is a mixture design?

5.5.4.5.

Treating mixture and process variables together

Options for setting up experiments for processes that have both standard process variables and mixture variables

Consider a mixture experiment consisting of q mixture components and k process variables. First consider the case in which each of the process variables to be studied has only two levels. Orthogonally scaled factor settings for the process variables will be used (i.e., -1 is the low level, 1 is the high level, and 0 is the center point). Also assume that each of the components x_i can range from 0 to 1. The region of interest then for the process variables is a k-dimensional hypercube.

The region of interest for the mixture components is the (q-1)-dimensional simplex. The combined region of interest for both the process variables and the mixture components is of dimensionality q - 1 + k.

Example of three mixture components and three process variables

For example, consider three mixture components (x₁, x₂, x₃) with three process variables (z₁, z₂, z₃). The dimensionality of the region is 5. The combined region of interest for the three mixture components and three process variables is shown in the two figures below. The complete space of the design can be viewed in either of two ways. The first diagram shows the idea of a full factorial at each vertex of the three-component simplex region. The second diagram shows the idea of a three-component simplex region at each point in the full factorial. In either case, the same overall process space is being investigated.

Diagram showing simplex region of a 3-component mixture with a 2^3 full factorial at each pure mixture run

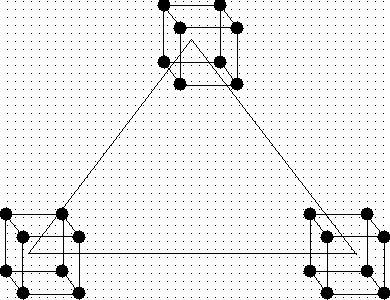
FIGURE 5.13: Simplex Region of a Three Component Mixture with a 2³ Full Factorial at Each Pure Mixture Run

Diagram showing process space of a 2³ full factorial with the 3-component simplex region at each point of the full factorial

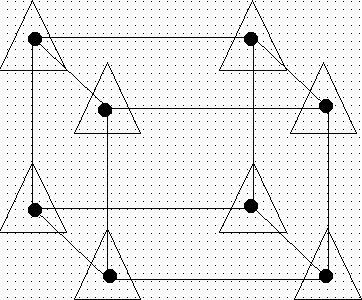
FIGURE 5.14: Process Space of a 2³ Full Factorial with the Three Component Simplex Region at Each Point of the Full Factorial

Additional options available

As can be seen from the above diagrams, setting up the design configurations in the process variables and mixture components involves setting up either a mixture design at each point of a configuration in the process variables, or similarly, creating a factorial arrangement in the process variables at each point of composition in the mixture components. For the example depicted in the above two diagrams, this is not the only design available for this number of mixture components with the specified number of process variables. Another option might be to run a fractional factorial design at each vertex or point of the mixture design, with the same fraction run at each mixture design point. Still another option might be to run a fractional factorial design at each vertex or point of the mixture design, with a different fraction run at each mixture design point.